As can be seen in [1], the end of the period-doubling cascade of any hyperbolic component hc is its Myrberg-Feigenbaum point MF(hc). What emerges from MF(hc) is what we denominate the shrub of the hc, due to its shape. If the shrub emerges from a primary hc (p/q), then we have a primary shrub, the shrub(p/q). If the shrub emerges from a secondary, tertiary,..., N-ary hc, then we have a secondary, tertiary ,..., N-ary shrub. Secondary, tertiary, ..., N-ary shrubs have 2, 3, ..., N subshrubs. As can be seen in [1], we can calculate the period of the representative hyperbolic component rhc of each one of the structural branches of the shrubs, as well as the preperiod and period of each one of the Misiurewicz points from which structural branches emanate (nodes) or where the shrub branches end (tips).
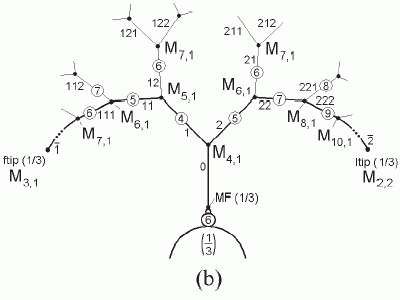
Shrub(1/3) is associated to a "decoration" formed by a node that is a Misiurewicz point from which three (p = 3) structural branches emanate. We can consider that the period-3 hyperbolic component 1/3, the origin of the shrub(1/3), is placed on the main branch 0, and that this branch 0 finishes on the main node 0, a Misiurewicz point M4,1. If we turn clockwise around the main node by following the branches 1 by 1 (because q = 1), we have the branches 0, 1 and 2 (or the hc's with periods 3, 4 and 5). At the end of each branch with a period-p rhc we have the Misiurewicz point Mp+1,1. So, at the end of the branch 1, whose rhc has period 4, we have the Misiurewicz point M5,1. If we turn clockwise around the M5,1 by following the branches 1 by 1, we have the hc's with periods 4, 5 and 6. Tips can also be found, being ftip(1/3) = M5,1. In such a manner we can find all the structural hc's and Misiurewicz points.
2. Shrubs(2/5)

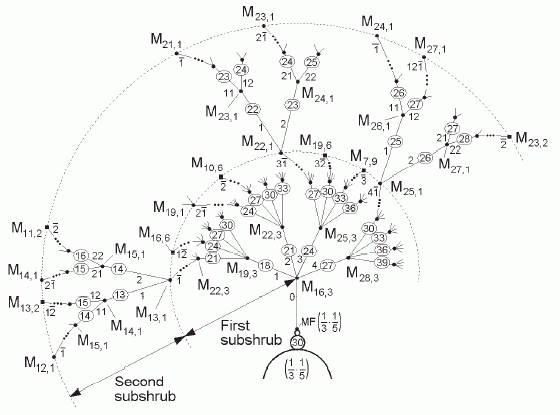
Shrubs(2/5) is associated to a "decoration" formed by a node from which five (p = 5) structural branches emanate. If we turn clockwise around the main node (but now by following the branches 2 by 2! because here q = 2) we have the branches 0, 1, 2, 3 and 4 (or the hc's with periods 5, 6, 7, 8 and 9). Again, at the end of each branch with a period-p rhc we have the Misiurewicz point Mp+1,1. For example, at the end of the branch 2, whose rhc has period 7, we have the Misiurewicz point M8,1. If we turn clockwise around this M8,1 by following the branches 2 by 2, we have the hyperbolic components with periods 7, 8, 9, 10 and 11. Here flip(2/5) = M5,1. In such a manner we can find again all the structural hc's and Misiurewicz points.
 Choose another shrub to "remove" labels.
Choose another shrub to "remove" labels.
[1] M.Romera, G.Pastor, G.Alvarez, and F.Montoya, "Shrubs in the Mandelbrot Set Ordering", accepted in International Journal of Bifucation and Chaos, 2002.